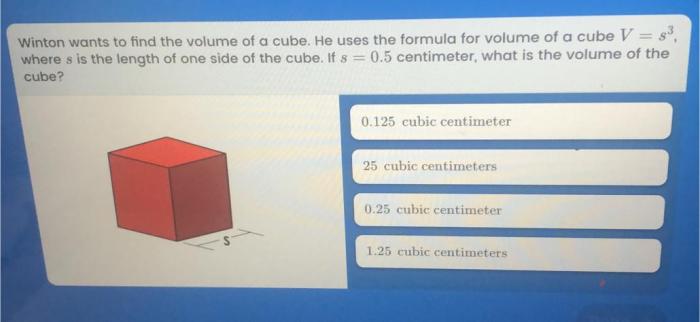
Winton wants to find the volume of a cube – Winton embarks on a captivating quest to unravel the enigmatic volume of a cube, setting the stage for an exploration that seamlessly blends mathematical precision with engaging storytelling. This discourse delves into the intricacies of volume calculation, providing a comprehensive guide for navigating the intricacies of this geometric concept.
As we embark on this mathematical odyssey, we will decipher the concept of volume, unravel the formula that governs the volume of a cube, and delve into real-world applications where volume plays a pivotal role. Together, we will unravel the secrets of Winton’s quest, empowering you with the knowledge to conquer any volumetric challenge that may arise.
Understanding the Concept of Volume: Winton Wants To Find The Volume Of A Cube
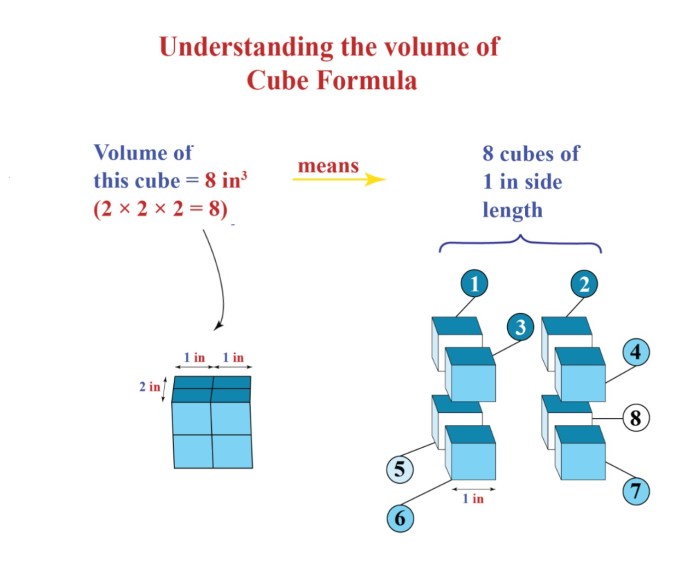
Volume is a crucial concept in geometry, representing the amount of three-dimensional space occupied by an object. It is a fundamental property that quantifies the size of objects and helps us understand their capacity, storage, and other practical applications.
For a cube, the volume is calculated using the formula: Volume = (Side Length)³, where the side length represents the length of each side of the cube.
Understanding volume is essential in various fields, such as architecture, engineering, and manufacturing, where accurate measurements and calculations are crucial for ensuring structural integrity, optimizing space utilization, and estimating material requirements.
Identifying the Given Information
To calculate the volume of a cube, we need to identify the given information, which typically includes the value of the side length.
For instance, if we are given that the side length of a cube is 5 centimeters, we have the following:
Side Length = 5 cm
This information is crucial for applying the formula and determining the volume of the cube.
Applying the Formula, Winton wants to find the volume of a cube
To calculate the volume of a cube using the given side length, we substitute the value into the formula:
Volume = (Side Length)³ = (5 cm)³
Performing the calculation, we get:
Volume = 125 cm³
Therefore, the volume of the cube with a side length of 5 cm is 125 cubic centimeters.
Interpreting the Result
The calculated volume of 125 cubic centimeters represents the amount of three-dimensional space occupied by the cube.
In practical terms, this means that the cube can hold or contain up to 125 cubic centimeters of material or substance.
Understanding the volume of the cube is crucial for various applications, such as determining the amount of liquid or gas it can store, estimating the weight of the cube based on its density, or calculating the surface area of the cube.
Answers to Common Questions
What is the formula for calculating the volume of a cube?
Volume = side length 3
How do I find the side length of a cube if I know its volume?
Side length = cube root of volume
What are some real-world applications of volume?
Volume is used in various fields, including architecture, engineering, and manufacturing, to determine the capacity of containers, calculate the amount of material needed, and design structures.